Rioux, MBA AM S 270408, 2008-2012
|
"In Rioux Recursive Architectures the various architectural subjects are broken down, repeated and reconstructed; their manipulated forms take on the appearance of x-rays, without actually seeing beyond their surfaces."
Neal Rockwell, 2012 |
Rioux, MBAmsgpres, 2008-2012
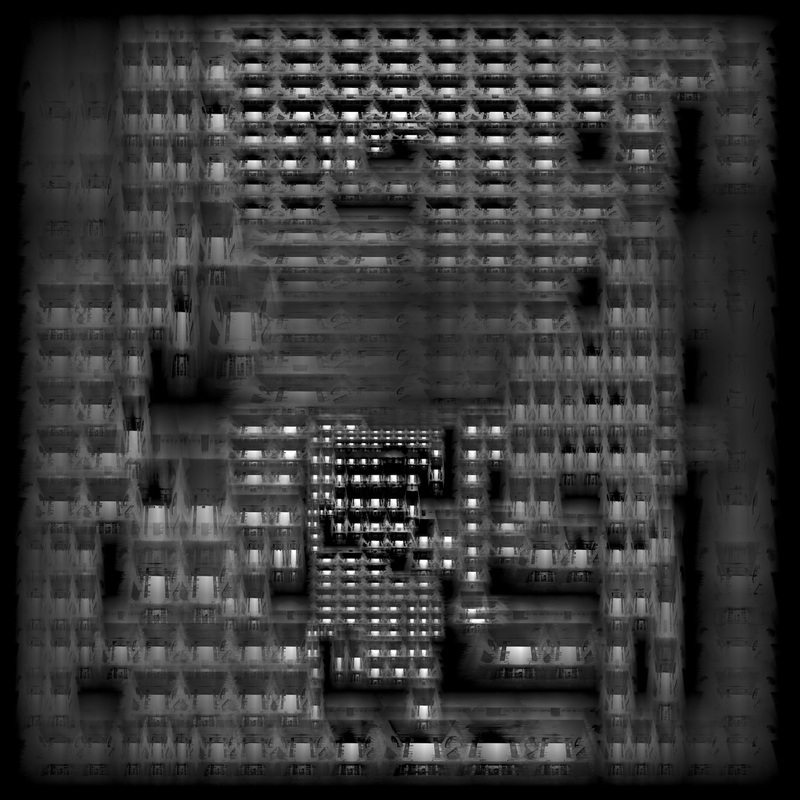
Recursive Architectures reevaluates the practice of architectural photography through reiteration and fragmentation of the subject.
Recursion is the process wherein, the following of a procedural set requires the reapplication of that procedure. It is the process of defining and breaking down something complicated in terms of a simpler base case. For instance all of the natural numbers can be defined by this recursive syllogism:
1 is in N (the set of all natural numbers),
if n is in N then 1 + n is in N, giving F(n + 1) = f(F(n)).
As well as a procedure to render complex things into simpler forms, recursion also allows certain simple and discreet structures to become infinite; for example, it is recursion in language (the ability to insert new clauses into pre-existing sentences) that transforms it from something finite into something unlimited.
Similarly, in the case of optical recursion – what happens when two mirrors face each other – the finite objects reflected in the mirror are replicated to infinity (or something close to it, owing to physical defects in the reflecting surface and the impossibility of perfectly parallel orientation).
In Rioux Recursive Architectures the various architectural subjects are broken down, repeated and reconstructed; their manipulated forms take on the appearance of x-rays, without actually seeing beyond their surfaces.
The building ceases to exist as a building – one can look inside and around the object but not at it directly. It becomes at the same time more abstract and more concrete through its repetition, but it ceases to exist as an independent object. Rather it becomes a symbol, and concurrently a convergence point that implies a relationship to a greater network.
Recursion is the process wherein, the following of a procedural set requires the reapplication of that procedure. It is the process of defining and breaking down something complicated in terms of a simpler base case. For instance all of the natural numbers can be defined by this recursive syllogism:
1 is in N (the set of all natural numbers),
if n is in N then 1 + n is in N, giving F(n + 1) = f(F(n)).
As well as a procedure to render complex things into simpler forms, recursion also allows certain simple and discreet structures to become infinite; for example, it is recursion in language (the ability to insert new clauses into pre-existing sentences) that transforms it from something finite into something unlimited.
Similarly, in the case of optical recursion – what happens when two mirrors face each other – the finite objects reflected in the mirror are replicated to infinity (or something close to it, owing to physical defects in the reflecting surface and the impossibility of perfectly parallel orientation).
In Rioux Recursive Architectures the various architectural subjects are broken down, repeated and reconstructed; their manipulated forms take on the appearance of x-rays, without actually seeing beyond their surfaces.
The building ceases to exist as a building – one can look inside and around the object but not at it directly. It becomes at the same time more abstract and more concrete through its repetition, but it ceases to exist as an independent object. Rather it becomes a symbol, and concurrently a convergence point that implies a relationship to a greater network.
|
Rioux, MBA Art-Map-2, 2012
|
Rioux, Innards 1, 2014
Rioux, Digital Cliff, 2010
Rioux, Op-2, 2007
Rioux, Recursive, 2009
Rioux, Turquoise a, 2015